How Can Rockets Fly in the Vacuum of Space?

Rockets are marvelous machines. Our generation is lucky to be able to witness rocket flights frequently, even if most of us have to follow new flights via live streams.

But have you ever considered how these rockets fly? How can rockets weighing tens of thousands of pounds overcome the grueling gravity of the Earth, both in our atmosphere and in the vacuum of space? How can they reach incredible velocities such as 27,000 kilometers per hour, or 17,000 miles per hour?

In this post, we will explore the basic principles behind the flight of these enormous and complex machines.

SpaceX

Newton's 3^rd Law: Law of Action-Reaction

All rocket engines work according to Newton's 3^rd Law, or the "Action-Reaction Principle". This law states that every force in the universe must have an equal and opposite reaction.

For instance, when you're standing, your weight due to the gravitational pull of our planet applies a force onto the Earth via your feet. If the Earth did not apply on you an equal and opposite reaction force, it would not be possible for you to stand still - and you would keep moving to the center of the Earth.

Another example is the Earth-Moon system. As our Earth pulls on its natural satellite (the Moon), the Moon also pulls our planet with the same force but with an opposing direction. Of course, since the mass of the Earth is much larger than the Moon's, the effect of this force on these two objects differ: The force applied on the Earth, even if it is exactly the same amount with the force applied on the Moon, does very little to our planet: For instance, it generates the tides - and it perturbs the orbit of our planet, but that's about it. On the other hand, that same amount of force applied on the Moon causes our satellite to stick to an orbit around the Earth.

Same thing is also true for you and the Earth: The force you apply is the exact same amount to the force that the Earth applies to you. But when you jump, you fall back on the Earth and the Earth does not fall on you. Because that force, regardless of its magnitude, has a much bigger impact on a small object like yourself than its impact on a humongous object like the Earth.

However, just to be clear, technically, the Moon does not orbit the Earth - but the Moon-Earth system orbits around a common center of gravity, which is known as the barycenter. This point is determined by the masses of the two objects in the system and is always closer to the more massive one.

In fact, as shown above, the barycenter of the Moon-Earth system lies inside the radius of the Earth and hence, it would appear to an outside observer that the Moon is orbiting around the Earth and not the other way around. The only effect that a detail-oriented observer would see is that the Earth does not follow a perfectly straight trajectory along its elliptical orbit, but it sways around the orbit like a drunk man trying to walk:

We are now ready to explore how rocket engines also adhere to this principle.

How Do Rockets Work?

The most common type of rockets involve an fuel tank that feeds liquid fuel to the engines to power the rocket. This fuel is mixed at a certain rate and pumped into the engine at a very high velocity. The flames that we can observe under a rocket is the fuel being burned by the engines. These flames will become important shortly.

Evrim Ağacı'ndan Mesaj

Neden Desteğe İhtiyacımız Var?

Aslında maddi destek istememizin nedeni çok basit: Çünkü Evrim Ağacı, bizim tek mesleğimiz, tek gelir kaynağımız. Birçoklarının aksine bizler, sosyal medyada gördüğünüz makale ve videolarımızı hobi olarak, mesleğimizden arta kalan zamanlarda yapmıyoruz. Dolayısıyla bu işi sürdürebilmek için gelir elde etmemiz gerekiyor.

Bunda elbette ki hiçbir sakınca yok; kimin, ne şartlar altında yayın yapmayı seçtiği büyük oranda bir tercih meselesi. Ne var ki biz, eğer ana mesleklerimizi icra edecek olursak (yani kendi mesleğimiz doğrultusunda bir iş sahibi olursak) Evrim Ağacı'na zaman ayıramayacağımızı, ayakta tutamayacağımızı biliyoruz. Çünkü az sonra detaylarını vereceğimiz üzere, Evrim Ağacı sosyal medyada denk geldiğiniz makale ve videolardan çok daha büyük, kapsamlı ve aşırı zaman alan bir bilim platformu projesi. Bu nedenle bizler, meslek olarak Evrim Ağacı'nı seçtik.

Eğer hem Evrim Ağacı'ndan hayatımızı idame ettirecek, mesleklerimizi bırakmayı en azından kısmen meşrulaştıracak ve mantıklı kılacak kadar bir gelir kaynağı elde edemezsek, mecburen Evrim Ağacı'nı bırakıp, kendi mesleklerimize döneceğiz. Ama bunu istemiyoruz ve bu nedenle didiniyoruz.

Destek Ol

As the fuel mixture combusts, the reaction gases exit the nozzle of the rocket at extremely high temperatures (3200°C or 5792°F) and insane velocities (~3,000 m/s, or ~6710 mph). This speed is needed for the rocket to be able to propel forward and overcome gravity.

NASA

Due to the Newton's 3^rd Law as explained above, as the "rocket system" (fuel tank, combustion chamber, etc.) applies a force on the exit gases, these gases apply an equal but opposite force on the rocket system. Since these gases are so lightweight, the combustion reaction causes them to fly out of the nozzle at incredible velocities. Even if individual gas particles are small, they are huge in numbers and every single one of those molecules push the rocket in the opposite direction.

Since the rocket is much heavier than the gases, a huge amount of inertia must be overcome by these shooting gases. This is why the rocket does not go as fast as the small molecules, but as the rocket roars, the rocket starts moving very slowly at first, but with a steady acceleration, allowing it to speed up as seconds pass.

This basic principle is so strong and reliable, that Saturn V, the most powerful rocket that has ever flown, was able to provide 34.5 million Newtons of thrust - an equivalent of 34,226 Boeing 747s!

Steven F.

As the rocket roars with fuels combusting, the pumps inside rocket engine (especially the "turbo pump", which is considered the "heart" of rocket engine) pumps fuel to the engine at very high velocities - and the pressure of the burnt fuel is also vital. In order to keep the rocket going, these huge pumps burn approximately 250 kg of fuel per second in Space X's Falcon 9 rocket and 788 kg of fuel per second in NASA's legendary Saturn V rocket.

How Can Rockets Fly in the Vacuum of Space?

This is a great time to talk about (and hopefully, correct) a common misunderstanding about how rockets fly. It is generally thought that rockets fly like how helicopters or most propelled systems (like drones) do, i.e. by pushing the air that they interact with. It is true that a helicopter needs air to push down so that it can generate lift in the opposite direction. But if this was true for our rockets, then none of our rockets could fly in space, since there are no molecules to push against to. In fact, Robbert Goddard, the (Western) father of modern rockets was ridiculed by the New York Times for proposing that machines could fly in the vacuum of space too - which eventually led to an apology by the newspaper, decades after the original article.

As we mentioned above, a rocket does not fly thanks to the atmospheric air pushed in the opposite direction. Instead, a rocket can fly thanks to the rocket pushing its burnt fuel out of its nozzle. In other words, a rocket generates its own gases to push against to, using chemical reactions (and converting liquids to high-velocity gases). So it is not like a person crouching down to jump even stronger - in that case, the person gains velocity by applying Newton's 3^rd law onto the Earth (the ground). A rocket's flight is more similar to a newly rising technology called "flyboards", where water or air is pushed at insane velocities to lift a person off of ground. In this case, the gases coming out of the jetpack allows the person to gain velocity in the opposite direction and lift off:

This is also how astronaut jetpacks work - by pushing internally pressurized air out of a nozzle to generate force in the opposite direction:

Thus, rocket engines can create thrust by pushing the gas that they create by burning their fuels, regardless of whether there is an atmosphere or not.

In fact, rockets work even more efficiently in the vacuum of space than they do here in Earth's atmosphere, since space is devoid of factors like other gases in front of the rocket which creates a drag force and slows the rocket's acceleration down. It is hard to grasp at first, but in fact, it is a more difficult process to overcome the drag forces generated in the atmosphere than to overcome the gravitational pull of the Earth. This is why Apollo missions (such as Apollo 17 shown below) could lift off the surface of the Moon with seemingly very little effort. The Moon has a gravity that is only 1/6^th of the Earth's (which is still a sizeable gravity), but it has close to zero air resistance due to a lack of atmosphere:

Conservation of Momentum

If you wanted to have a slightly more mathematical/physical understanding of how rockets work, let's freshen up your high school memories of learning about the conservation of momentum, i.e. the 1^st Law of Motion (or Newton's 1^st Law). Yes, in case you were wondering, rockets must follow all 3 laws of Newton and each law gives us a different insight about the flight of a rocket. We will skip it here, but briefly, Newton's 2^nd Law tells us how forces cause accelaration and that acceleration is what causes the rocket to speed up (remember, $F=ma$).

Momentum, or more specifically, linear momentum ($\vec{p}$) in this context, is a property of all objects in the Universe and it is calculated by multiplying the velocity ($\vec{v}$) of an object with its mass ($m$), as follows:

$\vec{p}=m\vec{v}$

Agora Bilim Pazarı

Albert Amca ile Maceralar Seti: Bilim Çocuk Kitapları (3 Kitap)

Çocuklarınıza bilimi sevdirecek, bilimsel kavramları öğretecek kitaplar arıyor ama bulamıyor musunuz? Bu seri tam size göre!

1. Albert Amca ile Zaman ve Uzay, Russell Stannard
2. Albert Amca ile Kara Delikler,Russell Stannard
3. Albert Amca ile Kuantum Macerası,Russell Stannard

Notlar:

1. Bu kitaplar, 9 yaş ve üzeri çocuklara uygun olacak şekilde hazırlanmıştır.
2. Bu kampanya, Kolektif Kitap tarafından Evrim Ağacı okurlarına sunulan fırsatlardan birisidir.

Devamını Göster

₺320.00

Satın Al Tüm Ürünler

• Dış Sitelerde Paylaş

Newton's 1^st Law states that, among other things, this momentum must be conserved at all times. This can be interpreted in that famous way: "An object at rest stays at rest and an object in motion stays in motion with the same speed and in the same direction unless acted upon by an unbalanced force."

So if there is no force to act upon an object, there should be no change in the velocity or direction of that object. But if there is a force on acting on an object, there must be a change in velocity and/or direction of that object.

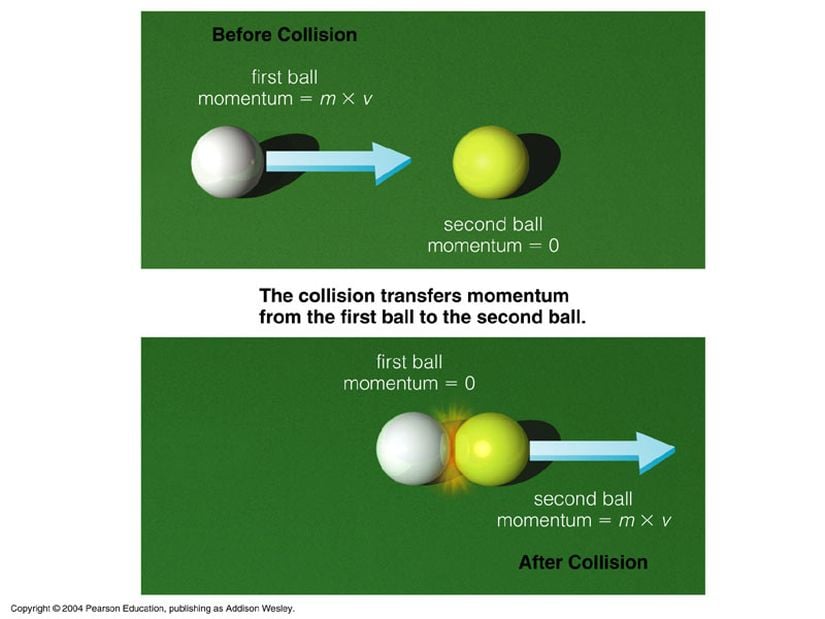
In Pool Balls

Think about a game of billiards: Let's say there are two balls at rest. Since both velocities of these balls are zero, then the net momentum of this system is zero.

Now, when we apply a force to the white ball through the pool cue, we have now added force to the system, therefore changing the velocity of the first ball, and therefore changing the momentum of that ball. As the ball moves toward the second ball we are aiming at, this first ball has a momentum of $m\vec{v}$, throughout its motion. The moment it makes impact with the second ball, this momentum needs to be conserved. As a result, if the first ball comes to a full stop after the impact, than the totality of its momentum ($m\vec{v}$) must have been transferred to the second ball. Since the second ball also have a mass of $m$, then it must have a velocity of $\vec{v}$, which is exactly equal to the velocity of the incoming (first) ball.

Pearson Education

In Rockets

Now let's apply the above principles to a stationary, upright rocket. It should be clear by now that the rocket's momentum must be conserved too. As the engine of the rocket burns liquid fuel, many very small molecules are pushed below the engine at incredible speeds in the flames. This creates a downward-directed net momentum which can be calculated by the summation of the mass of each gas molecule multiplied by its velocity with which it is propelled in the flames.

Pearson Prentice Hall

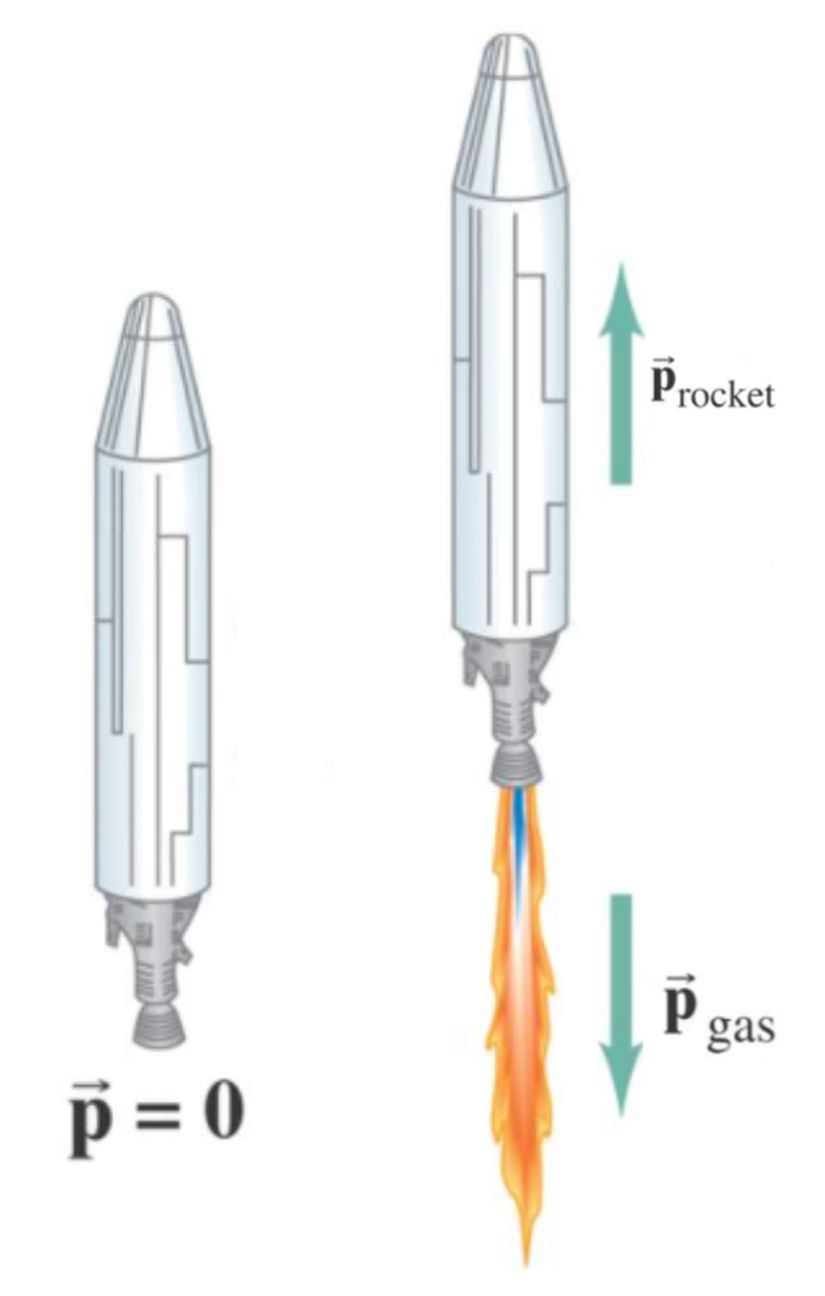
You can think of it this way: A single burnt fuel molecule is maybe quadrillions of times smaller, and hence, less massive than the rocket itself. Since their mass ($m$) is so small, regardless of the immense speeds ($\vec{v}$) at which they leave the rocket, the momentum ($m\vec{v}$) is still very minuscule. However, there are still quadrillions and quadrillions of these molecules leaving the rocket. Therefore, the summation of their momentum is extremely large, as each molecule's momentum is added on top of each other. And it is this total momentum that needs to be conserved, not just the momentum of a single molecule.

Since our rocket was stationary at first ($m\vec{v}=0$), this net momentum must be conserved. To do this, another momentum vector is required. This balancing momentum comes from the rocket, which rises in the opposite direction with the gases. Since the mass of the rocket ($m_{\text{rocket}}$) is extremely large, the total force applied to the rocket by the totality of atoms cause a very small velocity ($v_{\text{rocket}}$) at first. But it is a constant force, which slowly speeds the rocket up. And more importantly, that velocity generates an opposing momentum, conserving the net momentum of the system. This is how an enormous rocket can move away from our blue planet with magnificent flames.

Conclusion

Different companies have different rocket designs with unique and propriety systems, but they all operate with the same principles described in this article.

Wonderful Engineering

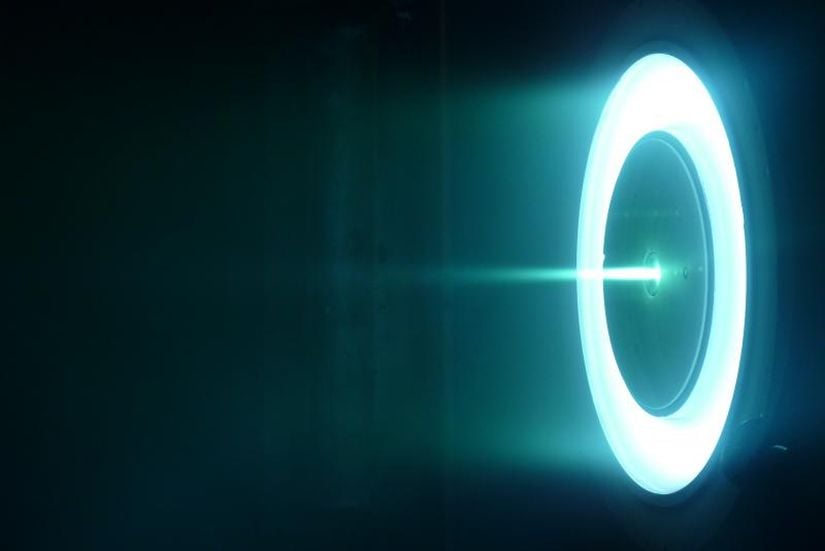
For instance, more recent technologies such as ion thrust systems ionizes gases with the electrical energy it captures from the Sun. Instead of burning fuel that we see in classic rockets that rely on chemical reactions, the ion thrust system ionizes gases that weigh less than fuel. The rocket uses electromagnetism to push the ions out of the rocket at very high velocities, creating the energy needed to propel the rocket rocket rapidly like a classic rocket engine. Thus, the rocket gains velocity in the opposite direction of the ions.

The ion thrust system shows promise in providing very long-termed thrust than a chemical rocket engine, allowing us to travel faster and longer in space. Although, in its current state the propulsion force is much, much lower than that of classic rocket chemical engines and the technology is not widely utilized in the rocket industry.