Threefold Epistemic Event Space (TEES): A Conceptual and Mathematical Framework
Physics
- Blog Yazısı
Abstract
This paper introduces a conceptual expansion of the Triple Event Space Theory (TEST) by framing it as a generalized Threefold Epistemic Event Space (TEES). The central hypothesis suggests that every observable event must be interpreted through at least three epistemically distinct possibilities: the observed outcome (ω₁), the unobserved but complementary counterpart (ω₂), and the transitional or uncertain state (ω₃). Building upon analogies such as coin flipping and quantum entanglement, the theory is enriched with a preliminary mathematical formalism. TEES attempts to bridge classical observation with quantum indeterminacy through a unified epistemic topology.
1. Introduction
The Triple Event Space Theory (TEST) proposes that any physical or cognitive observation inherently involves three possible epistemic regions. While traditional logic often reduces possibilities to binary outcomes (e.g., true/false, 0/1), TEES argues that such dichotomies are insufficient in describing the full scope of reality. Events unfold in ways that include not only a visible outcome and its complement, but also an intermediate state of ambiguity or transition.
2. Philosophical and Physical Foundations
The inspiration for TEES arises from the recognition that dualistic models struggle to explain phenomena in both macroscopic randomness (e.g., coin tosses) and microscopic quantum behaviors (e.g., particle spin). In both domains, a third category often exists—either as an ambiguous or unknown state (e.g., coin landing on its edge, superposition in quantum states) or as an emergent property of incomplete information.
3. Analogies and Illustrations
- **Coin Tossing**: Conventionally binary (heads or tails), the inclusion of a third possibility—edge—exemplifies ω₃, the transitional outcome.
- **Quantum Entanglement**: Before measurement, entangled particles exist in superposition. Upon observation (ω₁), the other particle’s state is instantaneously determined (ω₂), while their joint pre-measurement state remains ω₃.
4. Mathematical Representation
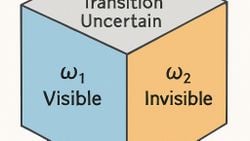
Let E be an event in a probability space Ω. Under TEES, E is defined by a triplet (ω₁, ω₂, ω₃), where:
Aslında maddi destek istememizin nedeni çok basit: Çünkü Evrim Ağacı, bizim tek mesleğimiz, tek gelir kaynağımız. Birçoklarının aksine bizler, sosyal medyada gördüğünüz makale ve videolarımızı hobi olarak, mesleğimizden arta kalan zamanlarda yapmıyoruz. Dolayısıyla bu işi sürdürebilmek için gelir elde etmemiz gerekiyor.
Bunda elbette ki hiçbir sakınca yok; kimin, ne şartlar altında yayın yapmayı seçtiği büyük oranda bir tercih meselesi. Ne var ki biz, eğer ana mesleklerimizi icra edecek olursak (yani kendi mesleğimiz doğrultusunda bir iş sahibi olursak) Evrim Ağacı'na zaman ayıramayacağımızı, ayakta tutamayacağımızı biliyoruz. Çünkü az sonra detaylarını vereceğimiz üzere, Evrim Ağacı sosyal medyada denk geldiğiniz makale ve videolardan çok daha büyük, kapsamlı ve aşırı zaman alan bir bilim platformu projesi. Bu nedenle bizler, meslek olarak Evrim Ağacı'nı seçtik.
Eğer hem Evrim Ağacı'ndan hayatımızı idame ettirecek, mesleklerimizi bırakmayı en azından kısmen meşrulaştıracak ve mantıklı kılacak kadar bir gelir kaynağı elde edemezsek, mecburen Evrim Ağacı'nı bırakıp, kendi mesleklerimize döneceğiz. Ama bunu istemiyoruz ve bu nedenle didiniyoruz.
- ω₁ ∈ Ω is the observed (visible) outcome.
- ω₂ ∈ Ω is the unobserved yet complementary outcome.
- ω₃ ∈ Ω is the uncertain/intermediate state.
We define a mapping φ: Ω → {ω₁, ω₂, ω₃}, with probabilities satisfying:
P(ω₁) + P(ω₂) + P(ω₃) = 1,
where ω₁ ∩ ω₂ = ∅, ω₁ ∩ ω₃ = ∅, ω₂ ∩ ω₃ = ∅.
Let the epistemic event space be a σ-algebra ℰ over Ω, partitioned as:
ℰ = {A ⊆ Ω | A = A₁ ∪ A₂ ∪ A₃, where Aᵢ ∈ {ωᵢ}}.
This extension allows us to encode uncertainty as a probability measure μ over the tripartitioned event space:
μ(A) = P(ω₁ in A) + P(ω₂ in A) + P(ω₃ in A)
5. Implications for Observation and Cognition
In TEES, any cognitive or physical measurement collapses ω₃ into either ω₁ or ω₂. The act of observation is thus seen as a transformation:
Φ: (ω₁, ω₂, ω₃) → ωᵢ, i ∈ {1,2}, such that ω₃ → ωᵢ.

This collapse parallels the quantum measurement problem, where wavefunction collapse results in a definite state from a range of possibilities.
6. Conclusion
The Threefold Epistemic Event Space model offers a compelling perspective to unify classical and quantum observations. By acknowledging the inherent triadic nature of all observable systems—observable, hidden, and uncertain—it challenges reductionist models and opens pathways for novel interpretations of indeterminacy in both physics and cognitive science.
References
[1] Wheeler, J. A., & Zurek, W. H. (1983). Quantum Theory and Measurement. Princeton University Press.
[2] Bohr, N. (1935). Can quantum-mechanical description of physical reality be considered complete? Physical Review, 48(8), 696.
[3] Fuchs, C. A., & Schack, R. (2013). Quantum-Bayesian coherence. Reviews of Modern Physics, 85(4), 1693.
[4] Zeilinger, A. (1999). A foundational principle for quantum mechanics. Foundations of Physics, 29(4), 631-643.
[5] Popper, K. (1959). The Logic of Scientific Discovery. Routledge.
[6] Dirac, P. A. M. (1930). The Principles of Quantum Mechanics. Oxford University Press.
[7] Heisenberg, W. (1927). Über den anschaulichen Inhalt der quantentheoretischen Kinematik und Mechanik. Zeitschrift für Physik, 43(3-4), 172-198.
- 0
- 0
- 0
- 0
- 0
- 0
- 0
- 0
- 0
- 0
- 0
- 0
Evrim Ağacı'na her ay sadece 1 kahve ısmarlayarak destek olmak ister misiniz?
Şu iki siteden birini kullanarak şimdi destek olabilirsiniz:
kreosus.com/evrimagaci | patreon.com/evrimagaci
Çıktı Bilgisi: Bu sayfa, Evrim Ağacı yazdırma aracı kullanılarak 21/05/2025 10:37:51 tarihinde oluşturulmuştur. Evrim Ağacı'ndaki içeriklerin tamamı, birden fazla editör tarafından, durmaksızın elden geçirilmekte, güncellenmekte ve geliştirilmektedir. Dolayısıyla bu çıktının alındığı tarihten sonra yapılan güncellemeleri görmek ve bu içeriğin en güncel halini okumak için lütfen şu adrese gidiniz: https://evrimagaci.org/s/20607
İçerik Kullanım İzinleri: Evrim Ağacı'ndaki yazılı içerikler orijinallerine hiçbir şekilde dokunulmadığı müddetçe izin alınmaksızın paylaşılabilir, kopyalanabilir, yapıştırılabilir, çoğaltılabilir, basılabilir, dağıtılabilir, yayılabilir, alıntılanabilir. Ancak bu içeriklerin hiçbiri izin alınmaksızın değiştirilemez ve değiştirilmiş halleri Evrim Ağacı'na aitmiş gibi sunulamaz. Benzer şekilde, içeriklerin hiçbiri, söz konusu içeriğin açıkça belirtilmiş yazarlarından ve Evrim Ağacı'ndan başkasına aitmiş gibi sunulamaz. Bu sayfa izin alınmaksızın düzenlenemez, Evrim Ağacı logosu, yazar/editör bilgileri ve içeriğin diğer kısımları izin alınmaksızın değiştirilemez veya kaldırılamaz.